Product Reliability With Emphasis on Field Data and Warranty Costs

 In the present age of global competition and mass production it is absolutely necessary to make accurate appraisal of product reliability, quality, and the satisfaction of customers at various stages of operation.  Incoming reports of incidents and successes on a product already in use provide important clues about its true nature.  It follows that we must make systematic analysis of dollar losses sustained as a result of product defects and operating failures which hurt our competitive standings. For example, American vehicle manufacturers have lost ground to foreign auto makers simply because countries like Japan, South Korea, and others have made systematic studies of loss functions involved in vehicle defects found in both laboratory tests and field tests. In fact, this whole approach, involving loss functions, has attained world-wide fame under the name of Taguchi, its Japanese promoter.

At DRI, we have for  over four decades promoted the so called Entropy Method of studying product reliability. The Dollar Loss Function idea of Taguchi is just another form of Entropy Analysis wherein defects are assigned dollar values instead of just being counted as occurrences (number of cases). See DRI Stat. Bulletin: Vol.18: Bul 3 and Bul.4.

The  CARS5A Software Package is based on such method for solving these type of field data.

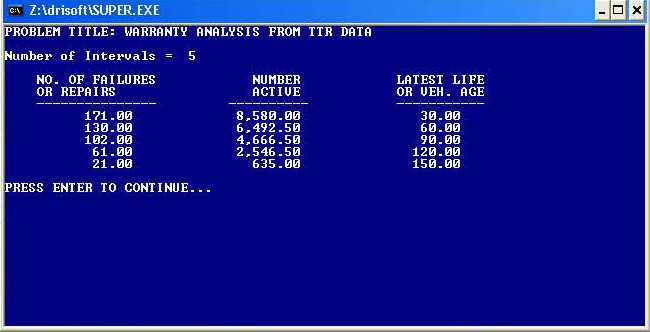
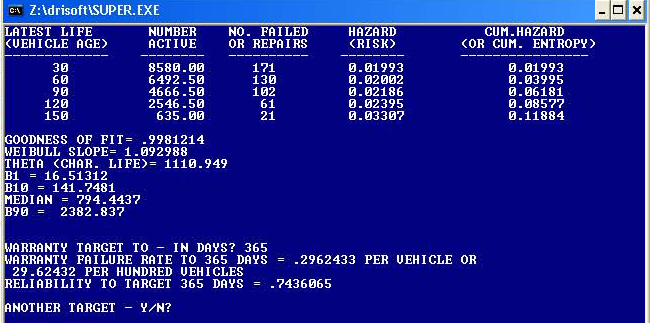
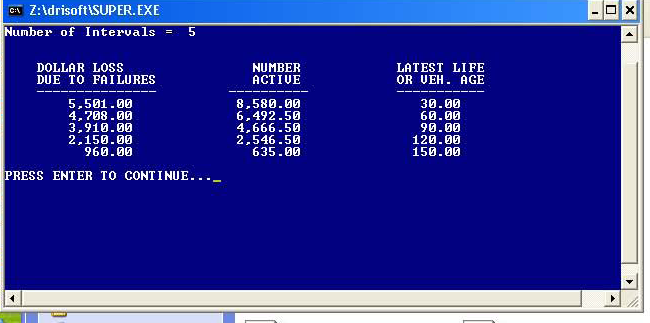
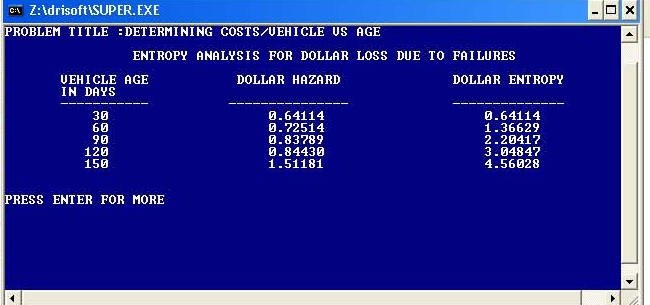
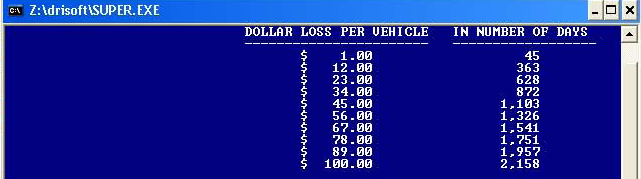
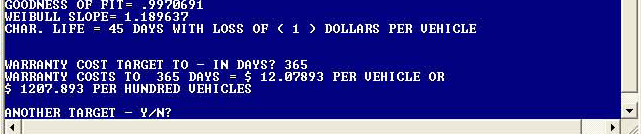
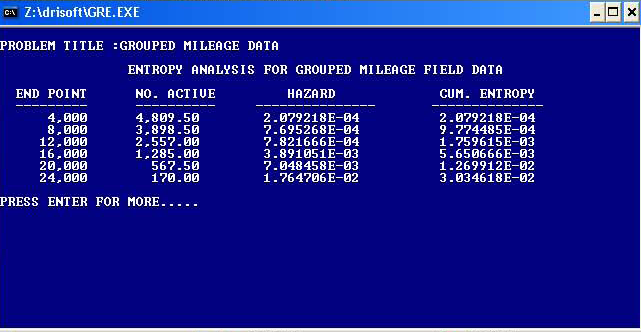
This type of analysis shown clearly that while a set of vehicle defects may appear to have an alarming total number of cases (like a B-10 life of only 142 days), the actual dollar losses per vehicle could be something trivial (like $12.08 per vehicle in the customer's first year of ownership), This all goes to show that in addition of counting repairs we should also count the costs involved.

 Example of Predicting Field Failure Rates by Suspended Set and Failed Set Technique via Entropy Method 

Table 1 - Failure Data on Vehicle Parts (Total in Field: 5,161)

Number Unfailed or Suspended = 5,143   Total Number of Failures  = 18

No. Suspended	No. Failed	Mileage Interval
702	1	0 - 4,000
1,116	3	4,001 - 8,000
1,562	2	8,001 - 12,000
975	5	12,001 - 16,000
451	4	16,001 - 18,000
337	3	20,001 - 24,000

 

This exactly the problem we faced with in the analysis of field failure, where the majority of the items are suspended items (i.e, unfailed), and are presented in a summarized form, in which various mileage intervals are listed together with the number of failed and unfailed vehicles in each mileage interval, it becomes necessary to formulate a special procedure based on the concept of Entropy for handling such sets of group data.  For this reason, the Entropy Method of estimating population parameters of Field Life has demonstrated superb value. This is all because the intrinsic ability of the Entropy concept in measuring the build-up of failure rates per vehicle.

 The Entropy Analysis of Grouped Mileage Field Data Computer Program of CARS5A  employed such concept.

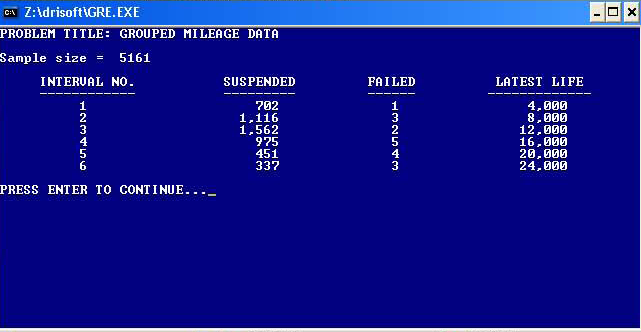
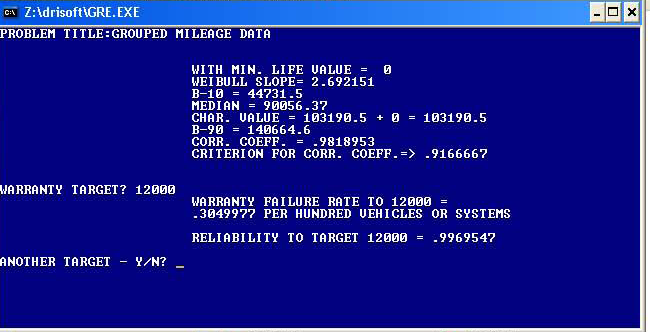
Note: Download our free "Weibull" Program to solve this example and see the difference between the Entropy Analysis of Group Mileage App.

This program indeed provides a more accurate estimates than the conventional Weibull Analysis.